Ultracold three-body recombination of fermionic atoms

H. Suno and B.D. Esry
Department of Physics, Kansas State University, Manhattan, Kansas 66506
Chris H. Greene
Department of Physics and JILA, University of Colorado, Boulder, Colorado 80309

Three-body recombination, a three-body collision process in which two atoms combine to form a bound state and the third carries away the binding energy, is an important loss mechanism for Bose-Einstein condensates. This process is also important in nuclear physics and in the chemical dynamics of combustion and gas-phase systems. Only recently have nonperturbative, quantum-machanical investigations been carried out [1,2,3,4]. Those investigations showed that the three-body recombination rate K3 of identical, spin-polarized bosons depends only on the two-body s-wave scattering length as.

Meanwhile, many groups [5,6,7] are carrying out experiments with ultracold trapped fermions. A degenerate Fermi gas (DFG) is expected to exhibit interesting behavior in its thermodynamics [8], collision dynamics [9], and the scattering of light [10,11]. The most intriguing prospect for a DFG is the potential to observe "Cooper" pairing, analogous to the Cooper pairing of electrons in a semiconductor. One of the limiting factors to such pairing is the loss of atoms by two-body or three-body inelastic collisions. To the best of our knowledge, ultracold three-body recombination of fermions has never been studied, in contrast to numerous theoretical investigations of boson recombination. So, we will present the first study on three-body recombination of ultracold fermions. In particular, a system of three identical spin-polarized fermions interacting via a sum of pairwise interactions will be considered.

Three-body recombination of bosons can be described in terms of the two-body s-wave scattering length as. In the case of fermions, however, the Pauli exclusion principle prohibits s-wave scattering of identical atoms, making p-wave collisions dominant at ultracold energies. When describing the three-body recombination of fermions, we use the the low-energy parameter Vp, the "p-wave scattering volume", defined by

Here, δp(k) is the p-wave scattering phase shift and k is the wave number. In our previous study [12], we found that for three identical fermions the permutation symmetry suppresses recombination as E2 near threshold. From this, by essentially dimensional analysis, we predict that K3 scales as |Vp|8/3. In contrast, the recombination rate for bosons scales generally as |as|4. To examine quantitatively the behavior of K3, we have performed nonperturbative calculations using the adiabatic hyperspherical representation.

We will present the results of our studies to date, including Figure 1. This figure shows the behavior of the three-body recombination rate K3 as a function of the scattering volume Vp at the fixed collision energy E=5 μK. We will discuss the various features of this curve, explaining them in physical terms. For instance, the |Vp|8/3 dependence predicted above is clearly seen as the straight lines in the plot of K33/8 versus Vp. The physics of two-body resonances, Stuckelberg oscillations, and three-body tunneling will be invoked in the discussion. Further, implications for and comparisons with experiments will be presented.

Figures:

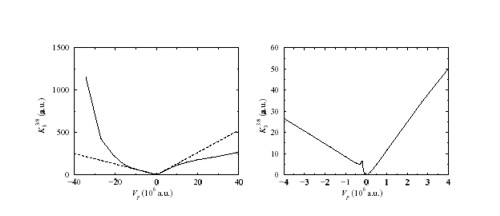
Figure 1: Three-body recombination rate as a function of the scattering for a wide range of the
scattering volume Vp at a fixed collision energy E=5 μK. The dotted line corresponds to the
|Vp|8/3 scaling. Right panel: Same as left panel, but with expanded Vp scale showing the scaling.

References:

[1] B.D. Esry, C.H. Greene, and J.P. Burke, Jr., Phys. Rev. Lett. 83, 1751 (1999).
[2] H. Suno, B. D. Esry, C. H. Greene, and J. P. Burke, Jr., Phys. Rev. A 65, 042725 (2002).
[3] E. Nielsen and J.H. Macek, Phys. Rev. Lett. 83, 1566 (1999).
[4] P.F. Bedaque, E. Braaten, and H.-W. Hammer, Phys. Rev. Lett. 85, 908 (2000);
E. Braaten and H.-W. Hammer, ibid 87, 160407 (2001).
[5] B. DeMarco and D.S. Jin, Science 285, 1703 (1999).
[6] K.M. O'Hara et al., Phys. Rev. Lett. 85, 160407 (2000).
[7] A.G. Truscott et al., Science 291, 2750 (2001).
[8] G.M. Bruun and K. Burnett, Phys. Rev. A 58, 2427 (1998).
[9] G. Ferrari, Phys. Rev. A 59, R4125 (1999).
[10] B. DeMarco and D.S. Jin, Phys. Rev. A 58, R4267 (1999).
[11] T. Busch, J.R. Anglin, J.I. Cirac, and P. Zoller, Europhys. Lett. 44, 1 (1998).
[12] B.D. Esry, C.H. Greene, and H. Suno, Phys. Rev. A 65, R010705 (2002).

This work was supported in part by the National Science Foundation.

Submitted to the Ugo Fano Memorial Symposium, July, 2002 in Cambridge, MA.

This abstract is also available in Postscript or Adobe Acrobat formats.

 
Return to do another abstract search of all our holdings.